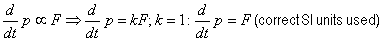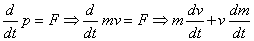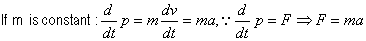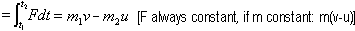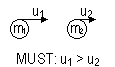DYNAMICS
Momentum
-all moving obj.s have momentum, more momentum > greater 'impact' when hits something
momentum, p = mass, m × vel,v > p = mv , p: units = Ns / kgm/s (p = linear momentum)
-same dirⁿ as vel, unbalance forces on obj > change in vel > change in momentum
Δp = p_final - p_initial = p_f - p_i

mass: property of a body to resist change in momentum
(inertia- tendency for a body to remain at rest / if moving, to continue its motion in a straight line, ie tendency to remain in state of motion)
weight: effect of gravitational field on a mass / force of pull of gravity on a mass

Newtons 1st law of motion: every obj continues in its state of rest or uniform motion in a straight line unless it is compelled to change that state by a net external force acting upon it (internal forces don't affect motion)
no force > no change in vel > no acc

Newtons 2nd law of motion: the rate of change of momentum of a body is proportional to the total force acting on it & occurs in the dirⁿ of the force




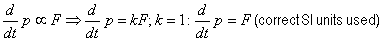
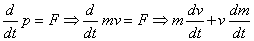
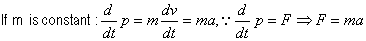
F=ma (F must be resultant force): 1N = force which gives 1kg an acc of 1m/s²

Newtons 3rd law of motion: if body A exerts a force o body B, then body B exerts an opposite force of the same size on body A (every action has an equal and opposite reaction) (2 bodies, 2 forces)

F = ma > F = m (Δv/Δt) > FΔt = mΔv (Δp = mΔv) > Δp = FΔt (mass & force: const)
Δp = FΔt = vector quan: impulse of a force (units = Ns) (dirⁿ of impulse = dirⁿ of Δp)

impulse = area under force-time graph


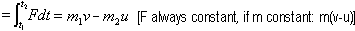
Conservation of momentum: total momentum of a system is constant provided no resultant external force acts on it
external forces: friction / gravity,.. (must be in closed system) Δp of one obj: equal and opposite of Δp of other obj

inelastic collision: loss of KE > heat / change in shape / sound,
(perfectly) elastic collision: no loss in KE
perfectly inelastic collision: not all KE lost, but obj.s stick together > move as 1
(all KE lost is possible if same mass + same vel in head-on collision)
glancing collision: one obj stationery

For perfectly elastic collisions, rel speed of approach = rel speed of separation
Verification of statement 'for perfectly elastic collisions, rel speed of approach = rel speed of separation'

rel speed of approach = u₁ - u₂
rel speed of separation = v₂ - v₁ [MUST: v₂ > v₁]
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂ (always)  (1)
m₁u₁² + m₂u₂² = m₁v₁² + m₂v₂²  (2)
From (1): m₁(u₁ - v₁) = m₂(v₂ - u₂)  (3)
From (2): m₁(u₁² - v₁²) = m₂(v₂² - u₂²)  (4)
(4)/(3): u₁ + v₁ = v₂ + u₂ > u₁ - u₂ = v₂ - v₁ (statement proven)


Back to 'A' level notes index

Back to notes index