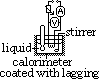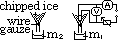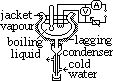THERMAL PROPERTIES OF MATERIALS
heat- form of energy transferred from a body at higher temp to one of lower temp by conduction, convection / radiation
amt of heat required to change temp of obj depends on
-mass of obj, -type of sub, -temp change
heat capacity, C- quan of heat required to raise temp of obj by 1K
C = ΔQ/ΔT (ΔQ: heat supplied, ΔT: temp rise)
C: J/K
specific heat capacity, c: amt of heat required to raise temp of unit mass by 1K
c = ΔQ/mΔT [J/(kgK)]
cm = C (specific heat cap × mass = heat cap)
Determining specific heat capacity of solid

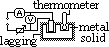
initial temp T, heater switched on: start timing & V, I noted, heater switched off: stop timing, record highest temp (assume no heat loss- heat gained by heater, thermometer, Hg ignored)
mcΔT = VIt
Determining specific heat capacity of liquid

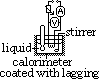
energy supplied = energy gained by [liquid + stirrer + calorimeter]
VIt = mcΔT + m_cc_cΔT

latent heat: temp const when subs changes phase (av KE of molecules const) (E supp used elsewhere- not to raise temp)
spec latent heat of fusion: heat required to change unit mass of sub from solid to liquid phase w/o temp change
spec latent heat of vaporisation: heat required to change unit mass of sub from liquid to gas phase w/o temp change
melting: E used to mainly raise PE
boiling: E used to increase PE & to expand gas against atm pressure

Determining latent heat of fusion of ice

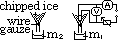
LH_fusion = heat supplied by heater
corrected m x L_f = VIt
corrected m = m₁ - m₂ (due to ice melting as a result of higher room temp)
use control exp w/o heater, find amt of ice melted over same time period
Determining latent heat of vaporization of water

water boiling: take mass & start timing
reach certain mass: switch off heater & stop timing
mL_v= Pt
more accurate method for determining latent heat of vaporization of water

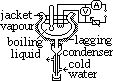
jacket of -vapour of liquid, -insulator (lagging)
heater switched on until whole apparatus at const temp
assume no heat loss: mL_v= Pt

Evaporation causes a cooling effect- explained in terms of latent heat or escape of high energy molecules
latent heat: energy needed to change liquid to gas > take heat for surrounding > cools surrounding
high energy molecules: loss of higher energy molecules (molecules w/ higher KE) > lower av KE of molecules > liquid cooler

Internal energy, U: sum of microscopic PE & KE of molecules
U = KE + PE
from kinetic theory; KE dependent on abs temp T, PE dependent on molecule sepn
gas: interatomic F- weak => U almost all KE
ideal gas: assume no interatomic F => U all KE => U dependent on temp
U_gas ^ when: - T ^, -WD on gas (compressed)
1^st law of thermodynamics: increase in int E of a system, ΔU is the sum of heat supplied to system, ΔQ & WD on system, ΔW
ΔU = ΔQ + ΔW


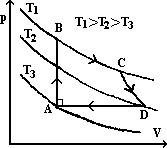
AB: p- increases, v- const; heat => no ΔE (no area under graph), ΔW = 0 => ΔU = ΔQ
BC: isothermal (const temp) expansion => more heat absorbed; ΔU = 0, T = const => WD by gas
CD: rapid expansion => no time for heat to leave gas => WD by gas = loss U
DA: isobaric (const p) change; gas loses heat => WD on it (compressed) & WD due to temp drop => U decreases
total cycle: ΔU = 0 (ends at beginning)

area under p-V graph = WD
ΔW = pΔV
ΔW = ∫pdV
ΔW: +ve: compressed, -ve: expands
ΔV = 0 => ΔW = 0


Back to 'A' level notes index

Back to notes index