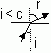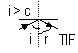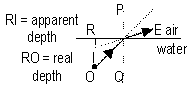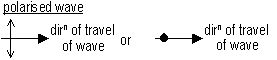WAVES
REFLECTION
Laws of reflection
-incident ray, normal & reflected ray all lie in same plane
-angle of incidence = angle of reflection

regular reflection(smooth surface) & irregular reflection

Properties of image
-same size
-upright
-virtual
-laterally inverted
-as far behind mirror as object is in front

REFRACTION
Laws of refraction
-incident ray, normal & reflected ray all lie in same plane
-for 2 given media, sin i/sin r = const

light; dense > less dense medium: refracts away from normal
light; dense > more dense medium: refracts towards normal

Refractive index, n = sin i/sin r = speed of light in medium 1/ speed of light in medium 2 = c₁/c₂ (from medium 1 > 2)
-depends on light colour (usually stated for yellow Na light)
₁n₂: n from medium 1 to medium 2 = c₁/c₂
if medium 1 = vacuum, ₁n₂ = absolute n (represented by n₂)
n_glass=1.5, n_water=1.33 (4/3), n_diamond=2.4, n_air=1.0003(regarded as negligible)
₁n₂=1/₂n₁, ₁n₃ = ₁n₂ x ₂n₃

Total internal reflection
occurs when light travels from (optically) dense > (optically) less dense medium

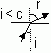
when ∠ r = 90°, ∠ i = critical ∠ (∠ c)

if ∠ i > ∠ c: light not refracted, only reflected at boundary

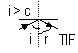
at i = c, ₂n₁ = sin i/sin r = sin c => ₁n₂ = 1/sin c
Uses of TIF: -reflecting prisms (no light loss, lasts long: no silver coat to wear away), -optical fibres (endoscope, TV, tel, comp signals)


Principle of reversibility of light

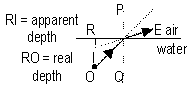
if light ray AE is reversed in dirⁿ to travel along EA, it will travel along AO n water
_air n _water = sin ∠ (PAE)/sin ∠ (OAQ) = (AR/AI) / (AR/AO) = AO/AI = real depth / apparent depth
(for small angles: OA → real depth, AI → apparent depth)





Wave motion

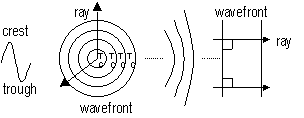
ray: lines of movement at right angles to wavefront
wavefront: lines joining particles that are in phase
crest: max upwards disp from equil pos (trough: min)
displacement: vector dist of a particle moved from rest position
amplitude: max displacement
phase difference: time difference (in radians) by which two oscillations differ from being in phase w/ each other
period: time for 1 complete oscillation
frequency: # of oscillations per unit time
wavelength: dist of 1 complete oscillation (smallest dist bet 2 pts in phase)
waves speed: speed w/ which crest (/trough) moves

Wave velocity = wavelength /time => v = λ/T => v = fλ

In SHM: total energy = ½ mω²A²
∴E ∝ A² => intensity ∝ (amplitude)², I =kA²
intensity: depends on energy passing through unit area in unit time
for waves (eg SHM), E associated w/ oscillation is ∝ A²

Transverse waves
-propagated by vibrations perpendicular to dirⁿ of travel of wave
-water wave, wave of plucked string, EM waves

Longitudinal waves
-vibrations // to dirⁿ of travel of wave
-partially displacement of particles (high density area: compressions, C + low density area: rarefractions, R)
(C & R move w/ speed of wave)


Polarisation


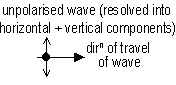
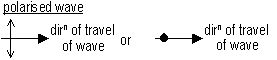
plane-polarised wave: a transverse wave whose particles vibrate in 1 plane only
polaroid: artificial material to causes plane-polarised light by allowing light of particular polarisation to pass
rotate 1 polaroid 90°: max bright > dark > darker > no light



Microwaves
shows T produces polarised waves => transverse waves emitted [longitudinal waves: no polarisation]



Amplitude of double polarised wave

Amplitude of initial electric vector = E_o
Amplitude of final electric vector = E = E_ocosθ
intensity ∝ amplitude² [E² = E_o²cos²θ]
∴intensity of b, I = I _ocos²θ [90°: I = 0, 0°, I = I _o]


Back to 'A' level notes index

Back to notes index